What type of organisms might be found in the tropical rainforest?

Unit Overview
Students will develop an understanding of how populations interact with each other within a community, discussing ideas concerning carrying capacity, competition, and interdependence. From there students will use models to explain the connection between genetic drift, natural selection, and speciation.
Standards
Next Generation Science Standards
Computational Thinking in STEM
- Data Practices
- Analyzing Data
- Manipulating Data
- Visualizing Data
- Modeling and Simulation Practices
- Using Computational Models to Find and Test Solutions
- Using Computational Models to Understand a Concept
- Computational Problem Solving Practices
- Troubleshooting and Debugging
- Systems Thinking Practices
- Investigating a Complex System as a Whole
- Thinking in Levels
- Understanding the Relationships within a System
Credits
Unit designed/developed by Dabholkar, S., Hall K., Woods P., & Bain C.
Acknowledgement
CODAP is developed and built by The Concord Consortium at https://codap.concord.org/
Lesson 7 is based on the lesson Evolution in Action: The Galápagos Finches Authored by Paul Strode for Howard Hughes Medical Institute based on data collected by Peter and Rosemary Grant, Princeton University.
This work is supported by the National Science Foundation (grants CNS-1138461, CNS-1441041 and DRL-1020101) and the Spencer Foundation (grant 201600069). Any opinions, findings, conclusions, and/or recommendations are those of the investigators and do not necessarily reflect the views of the funding organizations.
Underlying Lessons
- Lesson 1. Lesson 1: Introduction to Ecosystems and Models
- Lesson 2. Lesson 2: Population Dynamics
- Lesson 3. Lesson 3: Competition Between Individuals
- Lesson 4. Lesson 4: Competition Between Populations
- Lesson 5. Lesson 5: Genetic Drift
- Lesson 6. Lesson 6: Natural Selection
- Lesson 7. Lesson 7: Adaptive Radiation in the Galapagos
- Lesson 8. Lesson 8: Mechanisms of Speciation

Lesson 1 Overview
Purpose
To teach students to understand biological systems using individual- or agent-level behaviors and interactions.
Prerequisite Knowledge
- Food is a source of energy and building blocks for organisms
- Food is necessary for animals to survive
- Plants make their own food while animals and decomposers must consume other organisms
- Familiarity with food webs and trophic levels
Lesson Description
Students will use their prior knowledge of food webs to examine the specific ecosystem of Isle Royale. They will make predictions about how the populations of wolves and moose change over time, and attempt to construct a simple agent-based model based on the ecosystem.
Lesson Outline
- Introduction
- Think about differences between ecosystems broadly
- Think about interaction between populations broadly
- Think about Isle Royale broadly
- Predictions about wolf and moose populations
- Brainstorm rules for the model
- Revisiting predictions about wolf and moose populations
- Think about how and why models are useful
- Wrap-up
Lesson 1 Activities
- 1.1. Introduction
- 1.2. Interactions Between Populations
- 1.3. Studying Simpler Systems
- 1.4. Predicting Population Sizes on Isle Royale
- 1.5. Simulating Isle Royale
- 1.6. Revisiting Population Sizes on Isle Royale
- 1.7. The Purpose of Using Models
- 1.8. Summary
1.0. Student Directions and Resources
Students will develop an understanding of how populations interact with each other within a community, discussing ideas concerning carrying capacity, competition, and interdependence. From there students will use models to explain the connection between genetic drift, natural selection, and speciation.
1.1. Introduction
Modeling Interactions in Ecosystems
Purpose
What are the types of interactions that affect populations of organisms in an ecosystem?
Procedure
Brainstorm a list of organisms you might find in each of the forests that are pictured below.

Question 1.1.1
Question 1.1.2
What type of organisms might be found in the temperate deciduous forest?
Question 1.1.3
Why might you find different types of organisms in each forest?
1.2. Interactions Between Populations
Populations of organisms are often heavily influenced by their environments. However, other populations within an environment can also have a large influence. In the questions below, you will examine some of the ways in which populations can influence each other.
Question 1.2.1
How might a change in the size of one population affect the amount of resources available in the forest for other populations?
Question 1.2.2
Why might the change in the amount of resources available in the forest affect the size of other populations?
Question 1.2.3
How might a change in the size of a population indirectly affect the size of another population in an ecosystem? For example, how do you think a change in the population of mice in a forest might affect the population of moths?
1.3. Studying Simpler Systems
Background Information
Ecosystems are often difficult to understand because they usually include interactions between a large number of species. Isle Royale is different. It is a relatively simple island ecosystem, located 24 km from the shore of Canada in Lake Superior.

While there are many types of small animals on the island, and almost 20 types of mammals, only two species of the mammals that live on the island are relatively large. These are the wolves and the moose. On this island, wolves are the only predator of moose, and moose are essentially the only food for wolves.
To understand nature, it helps to observe an ecosystem where human impact is limited. On Isle Royale, there are no towns and people do not hunt wolves or moose or cut the forest. It is a very rare place on the planet where wolves, their prey, and the plants that support the prey are all left unharvested by humans. Isle Royale is remarkable, because nature runs wild there.
Moreover, because the wolves and moose on Isle Royale are isolated from the mainland by the surrounding water, they are unable to leave and new individuals are unable to come to the island except in very rare cases. Since scientists began observing the island in 1959, only one migration has occurred, which was a single wolf joining the island. Therefore, any population changes we might observe therefore are not the mere wanderings of wolves and moose to or from the island.
You're going to make some predictions about how the size of the wolf and moose population might change over time.
Question 1.3.1
Since moose can’t typically migrate on or off the island, what other factors might cause the size of the moose population to change from year to year?
Question 1.3.2
Since wolves can’t typically migrate on or off the island, what other factors might cause the size of the wolf population to change from year to year?
Question 1.3.3
Thinking about this community of wolves and moose in Isle Royale, do you believe that the size of the wolf population will change from one day to the next?
No
Question 1.3.4
Do you believe that the size of the wolf population will change from one month to the next?
No
Question 1.3.5
Do you believe that the size of the wolf population will change over the course of 30 years?
No
1.4. Predicting Population Sizes on Isle Royale
Scientists counted the number of wolves and moose on Island Royale every year starting in 1959. Here is the data for 1959 and for 2010.

In order to compare data from two populations, scientists plot the data using two different y-axes on the same graph. The y-axis on the left side of the graph below is the scale for the size of the wolf population. The y-axis on the right side of the graph below is the scale for the size of the moose population.
Question 1.4.1
Sketch the shape of the graph that you predict you will see for the size of the wolf population between 1959 and 2010.
In a different color, sketch the shape of the graph that you predict you will see for the size of the moose population between 1959 and 2010.

Question 1.4.2
What is the maximum number of moose that can be plotted on this graph according to the axis labels and intervals?
Question 1.4.3
What is the maximum number of wolves that can be plotted on this graph according to the axis labels and intervals?
1.5. Simulating Isle Royale
Based on the information you have been provided and the questions you have thought about, brainstorm a possible set of behavior rules for the wolves and moose and record them using the questions below. Don't worry about trying to come up with the 'right answer'. There are many possible answers which are valid, so just try to come up with a plausible, reasonable set of rules.
Discuss your rules with your classmates. Have some students behave as wolves and others behave as moose according to the rules established by the class, and think about what observations you can make about the ecosystem.
The model that we will end up using gives the same simple instructions to each type of organism, in this case moose, wolf, and plant.
What simple rules could you give to each of these types that would lead to a natural ecosystem? Think of simple commands like move, direction etc,,
Remember all organisms of the same type will follow the same rules.
Question 1.5.1
Please provide the rules for wolf behavior that your group came up with.
Question 1.5.2
How could you improve your rules (code) to more similarly mimic a real ecosystem?
1.6. Revisiting Population Sizes on Isle Royale
Based on your observations during the class simulation, do you have new thoughts about the population trends on Isle Royale?
Sketch the population trend you expect to see below.
Question 1.6.1
What did you notice about the wolf and moose populations and how they changed over the course of your model? Please provide at least one observation.
Question 1.6.2
Sketch the shape of the graph that you predict you will see for the size of the wolf population between 1959 and 2010.
In a different color, sketch the shape of the graph that you predict you will see for the size of the moose population between 1959 and 2010.

1.7. The Purpose of Using Models
Now that you have worked on developing a model of the Isle Royale ecosystem, you may have gained some insight into how and why models are useful in a variety of contexts.
Question 1.7.1
The Isle Royale ecosystem is very simple compared to many others available to scientists. What are some advantages to studying a simple ecosystem instead of a more complicated one?
Question 1.7.2
Why might studying an ecosystem with simple relationships between the organisms help us understand more complex places?
1.8. Summary
The original purpose of this activity was to answer: "What type of interactions affect populations of organisms in ecosystems?"
Question 1.8.1
What is the one big idea that you have discovered in this lesson?

Lesson 2 Overview
The Wolf-Moose Predation NetLogo model simulates the interactions between predator and prey within an ecosystem. These systems are looked at as being stable if these populations are able to maintain a relatively steady population over time, whereas an unstable system will result in the extinction in one or more of the populations.
Lesson 2 Activities
- 2.1. Population Dynamics
- 2.2. Introduction to the NetLogo Model
- 2.3. Introduction to the NetLogo Model
- 2.4. Stabilizing the System
- 2.5. Limited Resources
- 2.6. Complexity and Stability
- 2.7. Challenge
- 2.8. Assessing the Models
2.0. Student Directions and Resources
Students will develop an understanding of how populations interact with each other within a community, discussing ideas concerning carrying capacity, competition, and interdependence. From there students will use models to explain the connection between genetic drift, natural selection, and speciation.
2.1. Population Dynamics
Think back to the activity in Lesson 1 where you modeled a simple ecosystem consisting of predators (wolves) and prey (moose). As you may have observed during that activity, predator and prey populations oscillate over time. A graph of population data from Isle Royale is shown below as an example of these oscillations. Two scientists (Lotka and Volterra) modeled these oscillations using differential equations. A graph of these equations is provided for comparison.
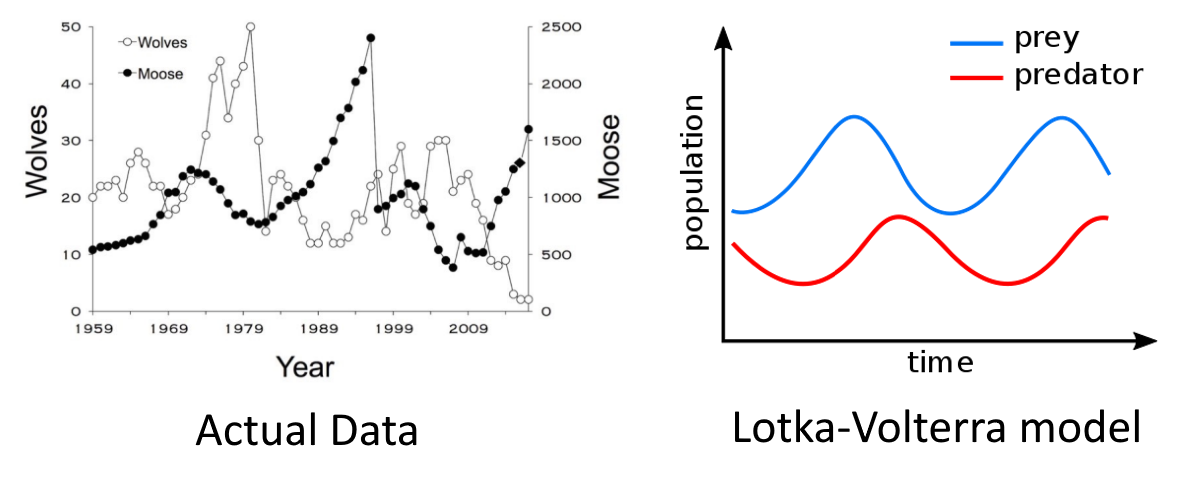
The equations used in this model state that these population oscillations are based on the birth and death rates of the predators and prey. The model also claims that the prey death rate and the predator birth rate are proportional to the number of prey caught by the predators.
Question 2.1.1
Based on the two graphs shown above, do you think the Lotka-Volterra equation-based model accurately describes how predator and prey populations oscillate? Why or why not? Describe the similarities and differences you see between the model and the actual data.
Question 2.1.2
Do you think the assumptions of the equation-based model are realistic? Can you think of any other factors that might influence the birth and death rates? If so, provide them below.
2.2. Introduction to the NetLogo Model
It is often helpful to understand how a model works before using it to investigate a phenomenon. In this activity, you will look at and interpret some of the underlying code that governs how the model runs. If you want to investigate beyond the questions in this activity, you can look at the complete code of any NetLogo model by clicking on the tab labeled 'NetLogo Code'.
All of the models you will see throughout this unit will have some things in common. For example, every model has individuals called agents which interact with each other in a variety of ways based on rules that govern their behaviors. Also, in every model, time moves forward in short steps called ticks. At the end of each tick, the model uses the agents' rules to calculate what the state of the model will be in the next tick. This means that to understand how the model works, it will be important to understand how the agents behave.
This model has two main types of agents, which are wolves and moose. The questions below will address how each of them behaves.
Question 2.2.1
This is a segment of code from the model which governs how wolves behave. Each wolf has an internal stash of energy, which is related to how healthy it is.
This code will be used every tick by each wolf. The gray text is a comment describing what the line of code does, but it has no effect on how the code works.

Think back to the rules for wolf behavior that your class created in the previous lesson. How do you think the rules represented in this code compare to the rules you came up with?
Question 2.2.2
This is a segment of code from the model which governs how moose behave. This code will be used every tick by each moose. It is similar to the code describing how wolves behave, but is slightly more complicated.

The model version variable can be set to either "moose-wolves" or "moose-wolves-plant". If it is set to "moose-wolves-plant", then the model will include grass as a renewable food source for moose and the code in the following brackets (lines 57, 58, and 59) will run. If it is set to "moose-wolves", then that code will not run. Based on this code, what do you think will change about how moose behave if grass is included or not included?
Question 2.2.3
Now you will explore the NetLogo Code tab. Both of the pieces of code from the previous questions use a function called MAYBE-DIE. Click on the tab below the model labeled 'NetLogo Code' and find the function called MAYBE-DIE (the first line of the function is 'to maybe-die'). How does this function work? What would cause a wolf or moose to die?
2.3. Introduction to the NetLogo Model
There are two main variations to this model that you will be working with.
In the first variation, wolves and moose wander randomly around the landscape, while the wolves look for moose to prey on. Each step costs the wolves energy, and they must eat moose in order to renew their energy. If wolves are unable to catch enough moose , they will die. At each time interval both wolves and moose have a fixed probability of reproducing, depending on the corresponding sliders. This form of the model follows the same assumptions as the Lotka-Volterra equation-based model described earlier.
Basics of the model
-
Make sure to select the "moose-wolves" option in the model version dropdown menu.
-
Click the SETUP button. You can click this button at any time to reset the simulation to its initial settings.
-
Press the GO button to begin the simulation. If you press the GO button while the model is running, this will pause the simulation.
-
Look at the monitors to see the current population sizes.
-
On the top of the world view you will see the word “ticks” with a number next to it. Each tick represents a unit of time passing by.
-
Look at the POPULATIONS plot to watch the populations fluctuate over time.
The behaviors of these organisms are influenced by changing the values of the green sliders. Go ahead and mess around with the model and sliders to get used to interacting with it.
Before answering the questions, let the simulation run for at least 300 ticks and observe the interactions of the wolf and moose over that time.
Question 2.3.1
Explain what each feature of the plot represents:
Question 2.3.2
When the lines on the graph intersect the first time, how many moose are present? You can hover your cursor over the lines on the graph to get exact numbers.
Question 2.3.3
Which of the populations increase first? Explain why you think this might be the case.
Question 2.3.4
Looking at the graph, do the peaks (highest point) of the animal populations overlap? If not describe what you see.
Question 2.3.5
A stable system will tend to have a relatively steady population over the course of time, while an unstable system will eventually result in the extinction of one or more of the populations. Would you describe this as being a stable or unstable ecosystem? Explain.
2.4. Stabilizing the System
As stated earlier, a stable system will tend to have a relatively steady population over the course of time, and an unstable system will eventually result in the extinction of one or more of the populations.
Your challenge at this point is to turn the "moose-wolves" ecosystem (which is based on the Lotka-Volterra equation-based model) into a stable system that allows for continuous generations of both wolves and moose for at least 300 ticks.
The variable "moose-wolves" must be selected in the model version dropdown menu.
You should only spend about 5-10 minutes on this activity. Don't worry if you aren't able to find a way to stabilize the system. Whether you stabilize it or not, describe your thought process by answering the questions below.
Question 2.4.1
Which specific variable(s) did you change and how did you change them?
Question 2.4.2
Explain why you made these changes. How do you think these changes helped to stabilize the ecosystem?
2.5. Limited Resources
In the previous activity, you probably weren't able to find a way to stabilize the ecosystem for 300 ticks. That is perfectly fine! In fact, it is not possible to make that ecosystem stable over long periods of time. In order to do that, there need to be some changes to the model itself, which you will explore next.
The second variation includes plants in addition to wolves and moose. The behavior of the wolves is identical to the first variation, however this time the moose must also eat plants in order to maintain their energy, and if they don’t they will die. Plants that are eaten by moose will regrow after a fixed amount of time depending on the plant regrowth time slider.
Select "moose-wolves-plant" in the model version dropdown menu. Keep all other settings the same.
Hit "setup" and "go" to start the simulation. Let the simulation run for 300 ticks before pausing the simulation by hitting "go" again and answering the questions.
Question 2.5.1
Explain the difference to the ecosystem when plants are present vs. absent.
Question 2.5.2
Explain how plants indirectly affect the population of wolves. Use the simulation to help explain your claim.
Question 2.5.3
Describe the relationship between the moose and plant populations over time. Be as detailed as possible in your description.
Question 2.5.4
Would you describe this ecosystem as stable or unstable? Support your choice.
Question 2.5.5
What do you think would happen if another moose predator was added to the habitat? Describe the effect on the moose as well as the wolf population.
2.6. Complexity and Stability
Question 2.6.1
In the second version of the model, there is a third type of organism (plants) in the ecosystem. This makes it a more complicated system, since there are more possible interactions between types of organisms. Were you surprised that making the model more complicated made it more stable? Why?
Question 2.6.2
Why do you think making the model more complicated made it more stable?
2.7. Challenge
Find three different ways that you can manipulate the simulation so that both populations die off without changing the initial population of either wolves or moose. When you figure this out, describe the variable(s) that you changed for each situation, and then explain why you think both populations were not able to survive.
Make sure to select "moose-wolves-plant" in the model version dropdown menu.
Question 2.7.1
Situation 1
Question 2.7.2
Situation 2
Question 2.7.3
Situation 3
Question 2.7.4
Describe your general approach to making the ecosystem fail.
2.8. Assessing the Models
At the beginning of the lesson, you were introduced to an equation-based model of population dynamics. In that model, population oscillations are based on the birth and death rates of the predators and prey, and the prey death rate and the predator birth rate are proportional to the number of prey caught by the predators.
Here you will evaluate and compare this equation-based model and the NetLogo model.
Question 2.8.1
List at least two limitations of using a model like these to make predictions about what could happen in the real world
Question 2.8.2
List at least two reasons why scientist might use a model like these.
Question 2.8.3
Based on your investigations, do you think that this equation-based model does a good job of explaining the phenomenon of population fluctuations? Why or why not?
Question 2.8.4
Based on your investigations, do you think the NetLogo model does a good job of explaining the phenomenon of population fluctuations? Why or why not?

Lesson 3 Overview
In this lesson, students are introduced to a participatory computer simulation where each student takes the role of an individual consumer (a bug) in an ecosystem. Students make predictions about various model runs and compare their predictions to the outcomes they observe. In one exploration they control the direction of movement of a bug, trying to gather as much food (grass) as possible in a variety of conditions. In another exploration they observe the outcome when many bugs move randomly and blindly around an ecosystem consuming food without any intentional control. Students recreate a physical representation of a histogram graph (of energy levels of bugs) from NetLogo and analyze characteristics of the population in the graph to draw comparisons between populations and individuals. Through discussion, the teacher helps build consensus about what they discovered: Competition is an emergent outcome that results from 1) limited resources necessary for survival, 2) and unequal distribution of those resources throughout the ecosystem, 3) and from interactions (intentional or unintentional) that always are occurring between each individual and their environment. In their homework students address the difference between intentional and unintentional competition further. They critique the modeling assumptions used in the computer simulation. They describe the variation in local resource availability for individuals in the computer model. They calculate how changes in the amount of grass or amount of bugs in would change the average amount of grass per bug in the ecosystem and they identify that ecosystems with lower average grass per bug would have higher levels of competition than those with higher average amounts of grass per bug.
Lesson 3 Activities
- 3.1. Competition Between Individuals
- 3.2. Exploration 1
- 3.3. Build a class histogram
- 3.4. Exploration 2
- 3.5. Making Sense of Your Data
- 3.6. Discoveries
3.0. Student Directions and Resources
Students will develop an understanding of how populations interact with each other within a community, discussing ideas concerning carrying capacity, competition, and interdependence. From there students will use models to explain the connection between genetic drift, natural selection, and speciation.
3.1. Competition Between Individuals
Purpose
What causes competition between individuals in an ecosystem?
Overview
In class today, you will participate in a competition against your classmates. Understanding ways in which competition occurs between individuals in a population is necessary to understand how complex interactions lead to changes in populations over time.
Question 3.1.1
Give an example for when you intentionally competed against another person?
Question 3.1.2
In general it probably isn't difficult for you to come up with examples of intentional competition, but what might unintentional competition look like? This would be competition against another person that is not done on purpose.
3.2. Exploration 1
First, open up the HubNet Client 6.0.2 application. A window will pop up asking for you to enter a username (you can just use your name) and a "Server" which which is a string of numbers and decimal points (called an IP address) that your teacher will give you. Once you've entered both of those, go ahead and click enter.
Model Rules
Let's make sure you're familiar with how the model works. In this model, each student controls a single bug. By clicking on the view, you can change the direction your bug travels. The goal is to get your bug to eat as much grass as possible. In addition to student-controlled bugs, there can be "bot" bugs that just randomly wander and eat grass around the world.
This model uses a software package in NetLogo called HubNet. This allows many different students on many different computers to control a single NetLogo model. Your view focuses only on your bug. Only the teacher can see the whole model. After everyone has answered the questions below, your teacher will start the simulation.
Question 3.2.1
When a spot of green grass is eaten by your bug, what do you think you'll see happen in that spot?
Question 3.2.2
Where will the energy amount of your bug show up?
Question 3.2.3
Will everyone be able to get an equal amount of food in this environment? Explain your answer.
3.3. Build a class histogram
Record the energy of your bug at the end of the competition. Write this value on a post-it note. Then add your post-it note to the correct spot on the class histogram of all the bugs' energy levels.

Question 3.3.1
Sketch a general shape of the histogram. Mark where on the histogram your bug's energy value was located.

Question 3.3.2
Were all bugs in the ecosystem equally successful at finding food? Use data to support your claim.
3.4. Exploration 2
Question
If the bugs move randomly and blindly through the ecosystem (instead of being controlled by you), how do you predict the outcome of the competition will compare to the previous exploration?
Make a prediction
Question 3.4.1
How successful with the randomly controlled bugs be compared to your previous activity?
Question 3.4.2
How much variation will there be among the randomly controlled bugs compared to your previous activity?
Question 3.4.3
Explain the reasoning for your answer to the previous questions. Be sure to address both the average level of success and the amount of variation.
3.5. Making Sense of Your Data
Now the teacher will repeat the previous activity, but instead of the bugs being controlled by you and your fellow students, they will move randomly.
Choose one or two bugs to follow, and pay attention to how they affect and are affected by the bugs around them.
Question 3.5.1
Was your prediction about the histogram correct?
No
Question 3.5.2
How did the outcome of this competition compare to the previous ones?
Question 3.5.3
In the last exploration, bugs were not being controlled by you or anyone intentionally, but were moving about randomly. While viewing the interactions of the bugs what evidence did you notice suggesting that a competition still occurred?
3.6. Discoveries
The original purpose of this activity was to answer: "What causes competition between individuals in an ecosystem?"
Question 3.6.1
Based on the model, what do you believe is the answer to this question?
Question 3.6.2
Think back to Isle Royale, where we looked at how populations can affect the resources available to other populations. How is what you did today similar or different?

Lesson 4 Overview
Students are introduced to a new participatory computer simulation where each student takes of a critter designer. They design the movement behavior, reproductive behavior, and if their critter is a consumer or predator, and they release a critter into an ecosystem in an attempt to outcompete other populations of critters that other students release into the ecosystem. As a class they investigate whether they can create at least one species of critter, which outcompetes all other species all the time, even as the environmental conditions are changing. They discover that this is impossible. Through discussion, the teacher helps build consensus about how changes in the environmental conditions and interactions affected the success of their population, why different trait combinations have different competitive advantages (different fitness) for survival, and why no single “design” is optimal all the time in a changing environment. This discovery partially motivates the investigation of the evolution WISE project as a future unit of study. In their homework students learn about other major environmental changes that have occurred over the history of life on Earth. They describe why environmental changes would change the competitive advantage for a set of traits in an ecosystem. They predict whether variation in individual attributes would increase the likelihood or decrease the likelihood of some individuals form their population surviving for various populations.
Lesson 4 Activities
- 4.1. Introduction
- 4.2. Predators
- 4.3. Resources and Carrying Capacity
- 4.4. Invaders
- 4.5. Making Sense of Your Data
- 4.6. Exploration
- 4.7. Discoveries
4.0. Student Directions and Resources
Students will develop an understanding of how populations interact with each other within a community, discussing ideas concerning carrying capacity, competition, and interdependence. From there students will use models to explain the connection between genetic drift, natural selection, and speciation.
4.1. Introduction
Competition Between Populations
Purpose
How do populations affect each other in ecosystems?
Model Rules
In this model, a population of bugs are able to wander the world, eating grass as they go. As the bugs eat grass, they gain energy, and as they move they lose energy. If they run out of energy, the bugs will die, and if they gain enough energy, they will reproduce.
In this scenario, you will investigate how the bug population behaves with no outside influences.
Set the region % grassland to 100 in and the initial-birds to zero in both ecosystems before you begin.
Question 4.1.1
What unit of time do you think a tick might represent in this model?
1 minute
1 hour
1 day
1 week
1 month
1 year
Question 4.1.2
How long (number of ticks) does it take for the bug population to reach a stable size? What is going on in the model that makes you believe the population is stable?
Question 4.1.3
This stable size is called a carrying capacity. Please provide a rough estimate of the carrying capacity of the bug population in this situation. (try moving your cursor over the graph)
Question 4.1.4
In this environment, there are always some bugs dying and some bugs reproducing. However, the model shows us that after the bug population stabilizes, it stays mostly constant. What does this suggest about the average death rate and average birth rate of the bugs after the population stabilizes? How do they compare?
Question 4.1.5
When the bug population is low, why does it increase to the carrying capacity? It will be helpful to think about what factors might affect the birth and death rates of the bugs.
Question 4.1.6
When the bug population is high, why does it decrease to the carrying capacity? Again, it will be helpful to think about what factors might affect the birth and death rates of the bugs.
Question 4.1.7
How do you think the carrying capacity of the bug population will change when predators (birds) are added to the ecosystem?
It will stay roughly the same
It will decrease
4.2. Predators
Model Rules
In the next model birds will be introduced into the ecosystem. If a bird catches a bug it gains energy. As it moves it loses energy. A bird can die if it loses all of its energy. A bird can have offspring if it collects enough energy.

Make a Prediction
In the ecosystem you looked at in the previous activity, there were no predators.
Now, you will run an investigation about how introducing birds to the ecosystem affects carrying capacity and fluctuation size for the bug population. The model allows you to setup two ecosystems.
- On the left, setup an ecosystem that has no birds. On the right create an ecosystem that has birds.
- Determine values of other variables that you will keep constant. Ensure that all variable values are the same on the right and left side, except for the number of birds.
- Setup and re-run the model a few times until you feel like you have generated enough evidence to reliably claim that the carrying capacity for the bugs and size of fluctuation for the bugs are the same, slightly different or noticeably different in the two ecosystems.
Question 4.2.1
Estimate the carrying capacity of the bugs when birds are present.
Question 4.2.2
How did the carrying capacity of the bug population change when predators (birds) were added to the ecosystem?
It stayed roughly the same
It decreased
Question 4.2.3
In the previous activity, you saw how carrying capacity can be the result of balancing birth rates and death rates in a population. Based on this, explain your observations about what happens to carrying capacity when birds are present.
Question 4.2.4
The presence of birds affects one or both of the birth and death rates. Why do you think the bug population settles into a new carrying capacity in the presence of birds? Why don't they just keep increasing or decreasing? Think about your answer to the previous question.
4.3. Resources and Carrying Capacity
Now, you will run an investigation about how different levels of resources in the ecosystem affects carrying capacity for the bug population. The model allows you to setup two ecosystems.
- Make sure that both ecosystems (left and right) have no birds.
- On the left, set up an ecosystem with 50% grassland. On the right, set up an ecosystem with 100% grassland.
- Determine values of other variables that you will keep constant. Ensure that all variable values are the same on the right and left side, except for the amount of grassland.
- Setup and re-run the model a few times until you feel like you have generated enough evidence to reliably claim that the carrying capacity for the bugs and for the bugs are the same, slightly different or noticeably different in the two ecosystems.
Question 4.3.1
How does the carrying capacity in the left ecosystem (50% grassland) compare to the carrying capacity in the right ecosystem (100% grassland)?
The two ecosystems have a similar carrying capacity
The left ecosystem has a lower carrying capacity
Question 4.3.2
In the previous activities, you saw how carrying capacity can be the result of balancing birth and death rates in a population. Based on this, explain your observations about the carrying capacity in the two ecosystems.
Question 4.3.3
Based on your previous answer, how do you think the carrying capacity of the bug population would change if another species that eats grass was added to the ecosystem?
It will stay roughly the same
It will decrease
4.4. Invaders
In this activity you will examine the effects of a competitor species on a population. The competitor species in this case is called invaders. The invaders eat grass, just like the bugs. The invaders do not eat bugs, and the bugs do not eat invaders.
-
Set the initial number of birds to zero and the grassland region to 100%.
-
Press SETUP and then press GO/STOP. As the model is running, press the LAUNCH AN INVASION button on one side, sometime between a time of 100 and 500 .
-
Run the model until it pauses on its own.
-
Record your observations below.
Question 4.4.1
Describe what happens in the model when you first add invaders to the ecosystem. Specifically, describe the effect on the bug population.
Question 4.4.2
Estimate the carrying capacity of the bugs when invaders are present.
Question 4.4.3
Try to find at least two different parameters to change that will result in the bug population dying out. Don't add predators or change the initial number of bugs. Briefly describe the approaches you used.
Question 4.4.4
Based on your understanding of resource availability and carrying capacity, why do you think invaders influence the bug population? How is this scenario similar to or different from the previous activity?
Question 4.4.5
Compare the total carrying capacity of the bugs and invaders in the left ecosystem to the carrying capacity of bugs in the right ecosystem. What do you notice about these values? Why do you think this is the case?
4.5. Making Sense of Your Data
You have separately examined the effects of predators and competing species on a bug population. However, in real ecosystems it is typical that both predators and competing species will be present at the same time. In the next scenario you will examine the behavior of the bug population when both of these are present.
Before moving on to that exploration, answer the questions below and think about your observations from the earlier activities.
Question 4.5.1
Did adding predators or adding invaders have a stronger effect on the carrying capacity of the bugs?
Invaders lower carrying capacity more
Predators and invaders lower carrying capacity just about the same
Question 4.5.2
Please try to explain this observation in terms of birth and death rates.
Question 4.5.3
How many ecological niches do you think are present in this ecosystem? Which species fall into each niche?
Question 4.5.4
How do you think these niches influence the competition between the populations?
4.6. Exploration
-
Choose an initial number of bugs, invaders, and birds to include in each environment. Make each at least 5.
-
Set the initial values for the amount of food bugs eat, the amount of food invaders eat, and the region amount of grassland to different combinations you decide. If you want to test the effect of changing one variable between the ecosystems, remember to make the other variables the same in both ecosystems.
-
Press SETUP and then press GO/STOP. As the model is running, press the LAUNCH AN INVASION button, sometime between a time of 100 and 500 .
-
Run the model until it pauses on its own.
-
Record your observations below. Try getting the two outcomes you selected by trying different combinations of values. If you can't find at least one set of values for each of the two outcomes you selected, then test some new possible combinations of values if time permits.
Design Your Investigation
Pick two of the following outcomes to generate in the model:
Outcome A: All three populations (bugs, invaders, and birds) die off.
Outcome B: The predator population dies off, but bugs or invaders survive.
Outcome C: The average predator population is above 100.
Try to find solutions that work consistently. If you run the model several times with your parameters, your chosen outcome should happen most of the time.
Question 4.6.1
What is the first outcome you will generate?
Outcome B: The predator population dies off, but bugs or invaders survive.
Outcome C: The average predator population is above 100.
Question 4.6.2
What parameters did you use to generate the first outcome?
Question 4.6.3
Why did this set of parameters accomplish your first outcome?
Question 4.6.4
What is the second outcome you will generate?
Outcome B: The predator population dies off, but bugs or invaders survive.
Outcome C: The average predator population is above 100.
Question 4.6.5
What parameters did you use to generate the second outcome?
Question 4.6.6
Why did this set of parameters accomplish your second outcome?
4.7. Discoveries
The original purpose of this activity was to answer: "How can we describe population size changes In ecosystem?"
Question 4.7.1
What is the one big idea that you have discovered in this lesson?
Question 4.7.2
How does the big idea you wrote down in the previous question inform or give new insights about what has happened in your case study?

Lesson 5 Overview
Students experiment with a population of bacteria growing in an environment with sugar as an energy source. The population of bacteria consist of different types represented with different colors. Different types of bacteria have different number of flagella; however, in this model there is no selective advantage of having more number of flagella. Students explore this model to investigate the phenomenon of genetic drift. They discover that even though there is no selective advantage of having more or less flagella, eventually only one type survives in the population. This happens because of statistical selection, also referred to as genetic drift.
Lesson 5 Activities
- 5.1. Get to know the model
- 5.2. Exploration 1: Investigate carrying capacity
- 5.3. Make a prediction
- 5.4. Test your prediction
- 5.5. Exploration 2: Investigate genetic drift
- 5.6. Understanding Randomness In Genetic Drift
- 5.7. Effect of carrying capacity on genetic drift
- 5.8. Discoveries
5.0. Student Directions and Resources
In this lesson, you will experiment with a population of bacteria. In this model the environment has sugar that bacteria use as an energy source. There are different phenotypes of bacteria in this population. Different types of bacteria have different number of flagella. Flagella are the appendages that allow bacteria move in specific direction. It's important to note that in this model there is no advantage for a bacterium to have more number of flagella.
Let's explore this model to investigate the phenomenon of genetic drift. Genetic drift is a mechanism of evolution. You will observe and learn about how the population evolves over time because of genetic drift. When you finish working through this lesson, we expect that you learn about genetic drift works as a mechanism of evolution. Let's get started!
5.1. Get to know the model
The model on this page is of a population of bacteria in an environment where sugar is an energy source. Before we explore the phenomenon of genetic drift, let's get to know this model first.
[* If the model is runs very slowly in your browser, use this GENETIC DRIFT MODEL for completing this lesson. You MUST have NetLogo installed on your computer to use the downloaded version of the model.]
Click 'SETUP' to initialize the model. Do NOT change any other parameters.
Make sure that the parameter values are as following:
#-phenotypes = 5
initial-#-bacteria-per-phenotype = 6
| left-resource -location | right-resource-location |
| around a central point | around a central point |
| left-resource-distribution | right-resource-distribution |
| 20% | 20% |
Run the model for 500 ticks for three times and answer the following questions.
Question 5.1.1
Note down the number of bacteria of the following types in the left region and in the right region in each simulation run.
Question 5.1.2
Explain why there may be variation in population sizes in the three trials.
Question 5.1.3
Change the resource distribution to 10% in the left and 80% in the right regions. Run the model. Describe the differences in the population growth in each region.
Question 5.1.4
In the previous question, you were asked to compare regions with 10% and 80% resource distributions. Before you ran your experiment, did you make sure that the resource locations were the same for both the left and right regions? Why might this be an important step in order to talk about the differences between 10% and 80% resource distributions?
Question 5.1.5
If, in the third question, you forgot to set the left and right region resource locations to the same thing (like "anywhere" or "horizontal strip"), go ahead and set them to the same thing and re-run your experiment. Using you new results, describe the differences in population growth between the two regions.
5.2. Exploration 1: Investigate carrying capacity
Do you remember what 'carrying capacity' means from the previous lesson?
Let's investigate how resource distribution influences the carrying capacity in this model.
Question 5.2.1
Write your definition of carrying capacity.
Question 5.2.2
Set resource-distribution to 10 %. Run the model. What is the carrying capacity of this environment?
50 to 100 bacteria
100 to 200 bacteria
more than 200 bacteria
Question 5.2.3
Set resource-distribution to 80%. What is the carrying capacity now?
50 to 100 bacteria
100 to 200 bacteria
more than 200 bacteria
Question 5.2.4
Explain how can you be certain that your carrying capacity numbers are correct.
Question 5.2.5
How does resource-distribution influence the carrying capacity in this model? Explain your reason.
5.3. Make a prediction
Imagine a bacterial population where there are only TWO TYPES to begin with. Let's make some predictions about how we expect such population will evolve.
Answer the following questions and then, on the next page, you'll test your predictions using the model.
Question 5.3.1
What do you expect to happen to the populations if you the model for a little while, like 5000 ticks?
Only one population will survive
Question 5.3.2
Which of the following do you expect to happen if you run the simulation for a really long time (for more than 100,000 ticks)?
Only one phenotype of bacteria will survive
5.4. Test your prediction
Set number of phenotypes to 2.
Set %-resource-distribution to 20%.
Run the model for 5000 ticks, using the speed slider at the top of the model to make it run faster if necessary. Note your observations in the space provided below the model. Then run the model for really long time (more than 100,000 ticks). Note your observations again.
Explain your observations by answering the questions below. Let's focus on the left region while you answer these questions.
Question 5.4.1
What were your observations when you ran the model for 5000 ticks? Explain those observations.
Question 5.4.2
What were your observations when you ran the model for really long time (more than 25,000 ticks)? Explain those observations.
A scientist has run this simulation for 25,000 ticks and has come up with the following plots. You could use these graphs below to answer this question.

Question 5.4.3
If you repeat the same computational experiment again, will you get the same results? Explain your answer.
5.5. Exploration 2: Investigate genetic drift
Genetic drift is the process of one type/color surviving without having any selective advantage. In this model, having higher number of flagella do not confer any selective advantage. We've observed that if we run the model long enough, we see only one type survive in the population.
So far we have explored the phenomenon of genetic drift, when there are only two types. Let's explore the phenomenon further when there are multiple types.
Let's focus on the left region while you answer these questions.
Answer question 14 below before you run the model to note down your predictions. Then run the model to test those predictions.
Question 5.5.1
Increase the number of types of bacteria to 6 or 7. How do you think the results will be different than when you had 2 types? Write your prediction. Do NOT run the model yet.
Question 5.5.2
Now run the model for at least 30,000 ticks. After you run the model, write your observations and compare those with your predictions.
5.6. Understanding Randomness In Genetic Drift
Genetic drift is sometimes referred to as statistical selection, because it is probabilistic or random. Let's understand the randomness in the phenomenon of genetic drift.
Question 5.6.1
Set % resource distribution to 20% to both the regions. Set the number of types to 5. Run the model three times until all phenotype have become extinct except for one.
Note your results in the table below. [If you are working in groups, you could write results from simulation-runs on different computers.]
Question 5.6.2
Describe your observations in the computational experiment you performed in the previous question. Explain your observations.
Question 5.6.3
What conclusions can be drawn from your observations?
5.7. Effect of carrying capacity on genetic drift
Let's investigate effect of carrying capacity on the process of genetic drift.
Set very low carrying capacity for the left region (10 % resource distribution) and very high carrying capacity for the right region ( 80 % resource distribution). In which region will you expect genetic drift to happen faster?
Question 5.7.1
What would be outcome of the simulation? Answer this question before you run the model.
Genetic drift will happen faster in the environment with low carrying capacity.
Genetic drift will happen at the same rate in the environment with low carrying capacity.
Question 5.7.2
Explain your reasons for the prediction.
Question 5.7.3
Write you observations after you run the model. Explain those observations.
5.8. Discoveries
The original idea of this lesson was to explore the phenomenon of genetic drift.
Question 5.8.1
Explain what genetic drift is in your own words.

Lesson 6 Overview
The teacher first introduces the new model. Students then design and perform computational experiments to explore how selective advantage because of different behaviors (due to a physical trait – flagella number) affect the outcomes of natural selection in population of virtual bacteria. Students present their initial results to the class and the class discusses possible explanations for why these different conditions yield different shifts in the distribution of trait variations from natural selection. Groups return to their experimentation and develop their explanations further, and report these out at the end of their experimentation. At the end of class, the teacher develops class consensus on the big ideas regarding the conditions necessary for natural selection and revises the scientific principle from the last lesson.
This lesson uses the same model as the previous lesson of a population of bacteria with different types. However, there are some important differences. In this model, there is an advantage of having higher number of flagella and there is cost associated to having flagella.
[Note: Computational scientists use variations of a model to study different but related phenomena. Though the model in this lesson looks similar to the one in previous lesson, it has important differences.]
Lesson 6 Activities
- 6.1. Natural Selection Model 1: Predation
- 6.2. Natural Selection Model 2: Environmental Conditions
- 6.3. Natural Selection Model 2: Make a prediction
- 6.4. Natural Selection Model 2: Test the prediction
- 6.5. Exploring effects of energy cost on natural selection
- 6.6. BehaviorSpace Experiment
- 6.7. Discoveries
6.0. Student Directions and Resources
In this activity students engage in simulated natural selection to discover how natural selection emerges from mechanisms: a) variation in heritable traits in a population and b) interactions in the environment give individuals with some variations a competitive advantage over other individuals. Another purpose of this lesson is to describe the outcome of natural selection as an increase in the proportion of individuals with advantageous heritable trait variations in a population over multiple generations.
6.1. Natural Selection Model 1: Predation
Let's look now at a different version of the bacteria flagella model that we used in the last lesson.
In this model, you are a predator. You can kill bacteria by moving the mouse cursor over and clicking.
Make sure to choose "none" from the VISUALIZE-PHENOTYPE menu, then set up the model and click on the button "run one minute experiment". The model will run for one minute. Try to kill as many bacteria as you can in that one minute. After the time is up, choose "flagella and color" from the VISUALIZE-PHENOTYPE menu. And click on "visualize" button.
Note your observations in the space provided below after you finish the experiment.
Question 6.1.1
Repeat the experiment twice. Write the number of bacteria of each variation at the end of the simulation run.
Question 6.1.2
What pattern do you notice in your results? Explain your observations.
6.2. Natural Selection Model 2: Environmental Conditions
Purpose
Find different environmental conditions that generate different trends in which number of flagella become more common over time due to natural selection. For example, what conditions favor lots of flagella? What conditions favor few flagella?
With a partner, discuss possible experimental conditions (in terms of %-resource-distribution or resource-location) that might generate these outcomes in the model.
Use the model to test your ideas with at least three environmental conditions.
[* If the model is runs very slowly in your browser, use this NATURAL SELECTION MODEL for completing this lesson. You MUST have NetLogo installed on your computer to use the downloaded version of the model.]
Question 6.2.1
How did the %-resource-distribution and/or resource-location affect the average number of flagella of the bacteria? Describe what you saw in each of the three conditions that you tested.
Question 6.2.2
Based on your observations from the model, why do you think this was the case?
6.3. Natural Selection Model 2: Make a prediction
Flagella help a bacterium to move and thus increase its chances of being near food before its eaten by other other bacteria. However, a bacterium has to incur some cost in terms of energy that it needs to spend to make and maintain a flagellum.
Make a prediction about which phenotype will survive after you run the model with limited resource conditions.
Question 6.3.1
Which of the following phenotypes will survive if you run the model (with 40% resource distribution around the central point) until only one phenotype survives?
You may choose more than one options if you think that the results will be different in each simulation run.
Phenotype with 2 flagella
Phenotype with 3 flagella
Phenotype with 4 flagella
Phenotype with 5 flagella
Phenotype with 6 flagella
Question 6.3.2
Explain the reason for your answer.
6.4. Natural Selection Model 2: Test the prediction
Set the given values for the following parameters:
| #-PHENOTYPES | 6 |
| INITIAL-#-BACTERIA-PER-PHENOTYPE | 8 |
| RESOURCE-LOCATION | 'around a central point' |
| RESOURCE-DISTRIBUTION | 40% |
Click setup and run the model until only one phenotype survives. Repeat the experiment 5 times and note your results in the table below. Make sure you uncheck the "view updates" box at the top of the model. This will still allow you to see the plots update, and will make the model run much more quickly.
Question 6.4.1
Fill in the table below:
Question 6.4.2
Describe any patterns you observe in the results of the previous computational experiment.
Question 6.4.3
| In this environment, the bacteria face selection against extremely small and extremely large numbers of flagella. This is an example of stabilizing selection, which reduces the amount of variation in a population. Stabilizing selection typically occurs when the environment isn't changing much. Why is there selection against a small number of flagella? Why is there selection against a large number of flagella? |  |
6.5. Exploring effects of energy cost on natural selection
In the previous experiment you must have observed that the phenotype with maximum number of flagella is not always successful in terms of its survival. This is because of the energy cost per flagellum.
Let's investigate the effect of the energy cost per flagellum on the process of natural selection.
Question 6.5.1
Set ENERGY-COST-PER-FLAGELLUM to 0.10. Make sure that all the other parameters are at their original default values. Run the experiment till 5000 ticks. What is the average number of flagella in the population?
Question 6.5.2
What will happen if you increase the ENERGY-COST-PER-FLAGELLUM and run the experiment again? Explain your answer.
Question 6.5.3
Design a series of computational experiments to systematically investigate the effect of ENERGY-COST-PER-FLAGELLUM on the process of natural selection. Describe your dependent variable and your independent variable.
6.6. BehaviorSpace Experiment
Download this Bacteria Food Hunt - Natural Selection NetLogo Model. You MUST have NetLogo installed on your computer to use the downloaded version of the model.
The software that you are using to explore the phenomenon of natural selection, NetLogo, has a feature called BehaviorSpace that can be used to conduct such experiments.
In this part you will use BehaviorSpace to conduct an experiment for investigating the effect of ENERGY-COST-PER-FLAGELLUM on 'avg. # of flagella' in the population after a certain time period.
- Go to "Tools" at the top of the screen and click "BehaviorSpace"
- Create a new experiment.
| Vary variables as follows |
|
| Repetitions | 5 |
| Run combinations in sequential order | Checked |
| Measure runs using these reporters | mean [phenotype] of bacteria |
| Measure runs at every step | Unchecked |
| Setup commands | setup |
| Go commands | go |
| Time limit | 5000 |
- The independent variable in this experiment will be the energy-cost-per-flagellum. In order to vary the energy-cost-per-flagellum, you will use the the BehaviorSpace tool. The format below is for varying this parameter systematically.
["energy-cost-per-flagellum" [energy-cost-in-the-first-run step-size energy-cost-in-the-final-run] ]
- Press OK and run the experiment.
- Select "Table output".
- Uncheck "Update view" and "Update plots and monitors" to run the experiment faster.
- The output will be in the form of a "csv" file. You will open it in Excel to analyze your data.
[* In case if you have problems using the BehaviorSpace experiment, use this data for your analysis.]
Question 6.6.1
We are using the code "mean [phenotype] of bacteria" to generate desired output after each run. Explore the 'code' tab of the NetLogo Model above and explain how this code will give us the desired value of the dependent (output) variable.
Question 6.6.2
In the spreadsheet file, create a plot of your dependent variable vs your independent variable. Upload the spreadsheet file here.
| File | Delete |
|---|---|
Question 6.6.3
Describe and explain your observations from the BehaviorSpace computational experiment that you just performed and analyzed.
6.7. Discoveries
How does natural selection change populations over time?
Question 6.7.1
In your words, describe the process of 'natural selection'. Explain how populations evolve because of natural selection.

Lesson 7 Overview
- A new trait might grant individual(s) a competitive advantage for survival and/or reproduction in an environment (an adaptation), or a competitive disadvantage, or neither.
- Advantageous traits tend to accumulate in populations over many generations yielding a population progressively better adapted to survive and reproduce in that environment over time.
Acknowledgement
CODAP is developed and built by The Concord Consortium at https://codap.concord.org/
This lesson is based on the lesson Evolution in Action: The Galápagos Finches Authored by Paul Strode for Howard Hughes Medical Institute based on data collected by Peter and Rosemary Grant, Princeton University.
This work is supported by the National Science Foundation (grants CNS-1138461, CNS-1441041 and DRL-1020101) and the Spencer Foundation (grant 201600069). Any opinions, findings, conclusions, and/or recommendations are those of the investigators and do not necessarily reflect the views of the funding organizations.
Lesson 7 Activities
- 7.1. How Do Species Emerge?
- 7.2. Introduction to Galapagos Finches
- 7.3. Intro to CODAP
- 7.4. What Causes Change in Finches?
- 7.5. More Finch Data
- 7.6. How Can Speciation Occur?
7.0. Student Directions and Resources
The purpose of this activity is to discover how the combination of mutations, natural selection, and environmental change generate progressively better-suited adaptations.
7.1. How Do Species Emerge?
Purpose
How do new species emerge?
Brainstorm
You know that many species that were alive in the past have gone extinct. Many of the species that are alive today did not exist at one point in the past.

Question 7.1.1
Explain how natural selection could help to explain how new species might emerge. Think back to some of the earlier concepts you went over.
Question 7.1.2
Can evolution occur without natural selection?
No
7.2. Introduction to Galapagos Finches
There are 13 species of finch on the islands, but they are at once both so similar and so diverse that they have provided a fertile ground for exploring evolution since Darwin’s 1835 visit. Darwin himself did not realize their role in explaining evolution until after ornithologists revealed the abundance of speciation to him.
The finches are proposed to have arrived on the volcanic islands from the South American mainland and are now considered part of the tanager family rather than the finch family. There are four genera recognized in the group, and the species occupy overlapping but distinct ecological niches. In the genus Geospiza, there are six species. In good times, they often eat the same foods, but in times of scarcity, each species has a specialized niche – large seeds, cactus fruits, etc. – on which they rely. Their mating behaviors, such as times and songs, differ greatly, maintaining the distinct species. The ecology of the different islands influences which species live on each island, and especially which species co-exist on an island. Gene flow between islands occurs with occasional immigrants depending on storms and the distance between islands.
For several decades, scientists have gone to the Galapagos islands to study the physical characteristics of the finches there. They recorded data on many traits including beak dimensions and weight. In this lesson you will explore some of that data to understand the processes underlying speciation and adaptive radiation.



Question 7.2.1
What do you think separates species from each other? In other words, what does it mean to be a 'different species' than another organism?
Question 7.2.2
How would you go about trying to distinguish one species from another?
Question 7.2.3
Why do you think the data the scientists collected might be useful for studying differences between species?
7.3. Intro to CODAP
Below is a data analysis tool called CODAP created by educators at the Concord Consortium. Using this computational tool, you will be able to delve deeply into the finch data mentioned on the previous page. When the page loads you will see the basic finch dataset with columns for sex, weight (g), beak length (mm), beak depth (mm). In CODAP we are able to interact dynamically with the data, allowing us to make connections and draw conclusions. We will use this set to answer several questions about these Galapagos finches.
Question 7.3.1
Use CODAP to fill out the following data table.
You can see the value of a data point by hovering your mouse over the point. Use this to find the minimum and maximum beak lengths.
Clicking on the data point will highlight the row in the data table.
Clicking on a graph will cause a toolbar to appear next to it. You can find and display useful information about the data in a graph using the ruler menu in that toolbar. Click the check boxes for median, mean, and standard deviation to display them on the graph. You can find their values by hovering your mouse over the display.
Question 7.3.2
If you click and drag to surround points on a graph, they will be selected on all current graphs. You can use this to hide points that you don't want to see. Sometimes CODAP responds slowly and will have a slight delay, so you may need to wait for it to catch up.
Click and drag to select all of the male finches. Then, on the histogram of beak length, use the eye menu to the right of the graph to hide unselected points. For clarity, you can also change the title of the graph to "Males" by clicking on the current title in the blue bar at the top of the graph.
What is the mean beak length for male finches?
Question 7.3.3
In order to visually compare two or more subgroups, it can be helpful to have multiple graphs. Sometimes the points on a new graph will not look the same as those on other graphs. You can change the appearance of the points on any graph using the paintbrush menu to the right of the graph.
In the upper left corner, click the "Graph" button to create a new blank graph. Drag the "Weight" column header from the table to the x-axis of the new graph to create a second histogram and title it "Females". Using the same method as before, hide all of the points on the new plot that aren't from female finches. Drag the "Weight" column header to the x-axis of the original graph to replace "Beak Length".
What differences do you notice in the graph of male finches vs. the graph of female finches? Be sure to mention characteristics like shape of the graph and median values.
Question 7.3.4
Another way to compare subgroups is to put categorical data on the y-axis. Close one of the two histograms and use the eye menu to show all points on the remaining graph. If you want, you can change the title for clarity. Then, drag the "Sex" column header to the y-axis of the histogram.
How does the group of finches of unknown sex compare to the male and female finches?
Question 7.3.5
Drag the "Beak Length" column header to the y-axis of the histogram to turn it into a scatter plot. If you still want an idea of how the male, female, and unknown sex finches compare, you can drag the "Sex" column header to the middle of the plot to change the color of each point to match the sex of the finch.
Based on this plot, what seems to be the relationship between weight and beak length in these finches?
Question 7.3.6
The data above comes from only one species of finch. Why do you think there is variation in the beak lengths and weights of these finches? Think back to some of the earlier lessons when you saw a graph like this.
7.4. What Causes Change in Finches?
The scientist that have collected this data have done so for over 40 years now, the first bar graph that you saw in section one contains finch data from 1973 -1981. Lets see what we can find if we look deeper into the data.
In this data set, "Last Year" is the record of the last year an individual finch was seen by the researchers. This typically means that the individual finch died during that year.
Use the methods you learned in the last activity to compare the finches that died during 1977 with the finches that survived 1977 and answer the questions below.
Question 7.4.1
What differences do you see between the group of finches that only lived until 1977 and the finches that lived to 1978 and beyond? Please discuss the position (i.e. mean, median) and shape (i.e. standard deviation, range) of the beak depth distributions in your response, along with any other information you think is relevant.
Question 7.4.2
|
The medium ground finch (Geospiza fortis) has a short, blunt beak which is adapted to picking up seeds from the ground. In 1976, seeds on the island were diverse and plentiful. During a drought in 1977, seeds became much harder to find. Once the finches had eaten all the small and medium-sized seeds, they had to turn to larger, spiny seeds that are hard to crack open. In your group come up with a reasonable hypothesis as to why there might be changes in how beak depths are distributed before and after 1977. Think about connecting past ideas like competition and natural selection. Be as specific as you can. |
|
Question 7.4.3
| During the drought, the beak depth with the greatest fitness increased, but the amount of variation in the trait did not. This is an example of directional selection. Directional selection is often the result of a change in environmental conditions. How does this compare to the stabilizing selection you saw in the previous lesson? |  |
7.5. More Finch Data
This CODAP frame has a much larger data set than those you have explored in the previous activities. To help you gain a better understanding of the finches in the Galapagos, there are many more physical traits to explore. Use the skills you developed in the previous activities to use CODAP to look at several traits and compare them across species and locations.
Not all of the traits were measured on each individual, so some traits will have more complete information than others. We will focus on a trait that has a lot of data points, beak height.
Question 7.5.1
Generally speaking, how are the different finch species similar or different?
For example, which species have similar ranges of beak height? Which species have different ranges of beak height?
Question 7.5.2
Generally speaking, how are the finches on different islands similar or different?
For example, you might think about whether the islands all have the same species, or whether the islands all have similar distributions of beak height.
Question 7.5.3
Generally speaking, are members of the same species on different islands different from each other? Give examples to support your answer.
Question 7.5.4
Look at the histogram of beak heights for all finches. There appear to be several peaks in it. Are there multiple species or islands represented in each peak? What does this suggest about the niches present in this ecosystem and the species that are in a peak together?
Question 7.5.5
Do the answers to any of these questions change if you look at another trait with many data points, like wing length or N-UBkL (another measure of upper beak length)? How?
7.6. How Can Speciation Occur?



Question 7.6.1
Scientists think the finches on the Galapagos are descended from finches that traveled from the mainland at some time in the past. One possibility is that one type of finch arrived at the islands and split into new species over time. Another possibility is that several species arrived at the islands. Which do you think is more likely? Why?
Question 7.6.2
Do you think the environments on each island are similar? How might they be different? It may be helpful to think about this in terms of ecological niches, and to think back to what you saw in the previous activity.
Question 7.6.3
Why do you think there are so many different species of finch on such a small group of islands? How might differences between the islands and their niches have affected the number of species?
Question 7.6.4
Come up with a story that describes the how the different species of finches could have developed in the Galapagos islands.

Lesson 8 Overview
Purpose: The purpose of this activity is to understand how new species can form from old species through the mechanisms of evolution covered so far in the unit (mutation, genetic drift, changes in environmental conditions, and natural selection).
Connection to previous activities: Students refer to the mechanisms of mutation (introduced in the last activity), genetic drift (from the activity before that), changes in environmental conditions and natural selection (from two previous activities), to develop the explanations for the outcomes in this activity.
Learning Performances
• Analyze data from a computer investigation applying concepts of statistics and probability to explain why adaptations for reproductive isolation can help reinforce specialized adaptations for survival for different niches within different gene pools in a population. [Emphasis is on analyzing shifts in numerical distribution of traits in a histogram and using these shifts as evidence to support explanations.]
Scientific Principles Discovered in This Activity:
• New species emerge from old species (a group of organisms that is capable of interbreeding only between each other to produce fertile offspring).
• Speciation can occur when specialization for survival in different niches is available to a population; this specialization opportunity can tend to reinforce adaptations that lead to greater reproductive isolation between those populations.
• Speciation can occur when geographic isolation leads to separate populations that through mutation and genetic drift, develop genes and corresponding traits that make descendent from each population less reproductively compatible with each other over time.
Description of the Lesson
The class revisits their definition of a species and discusses whether genetic drift alone could account for why new species emerge.
They then use a computer model of plants in an ecosystem to explore how speciation always could also emerge from a single population over time under certain conditions.
Through discussion, the teacher helps build consensus about why speciation might occur when mutation initiates the pathway to speciation, but natural selection and adaptation are the driving mechanisms that continue to reinforce the emergence of this outcome.
In the homework, they study examples of how speciation has been created in laboratory conditions with human intervention and contrast the mechanisms at work in real world ecosystems when new species emerge. And they read Darwin’s finches on the Galapagos Islands as a real-world example of adaptive radiation.
Lesson 8 Activities
- 8.1. Introduction
- 8.2. Predict
- 8.3. Looking at Flowering Time
- 8.4. Looking at Metal Tolerance
- 8.5. Predict
- 8.6. Test Your Predictions
- 8.7. Discoveries and Insights
8.0. Student Directions and Resources
The purpose of this activity is to understand how new species can form from old species through the mechanisms of evolution covered so far in the unit (mutation, genetic drift, changes in environmental conditions, and natural selection).
8.1. Introduction
Purpose
What are other ways that new species can form?
Brainstorm
Last lesson you looked at species formation over time based on geographic separation. Is this the only way that new species can form?
Question 8.1.1
Brainstorm a definition of a species based on your knowledge from the previous lesson.
Question 8.1.2
Besides geographic isolation, what other type of scenario could lead to new species being formed?
8.2. Predict
Your teacher will play this video for the class, but it is provided here for reference.
Question 8.2.1
What does the difference in color of the soil represent?
Question 8.2.2
How does the model show when flowering is occurring?
Question 8.2.3
What should occur when you change time-steps from days to years?
Question 8.2.4
The video discussed a scenario where neither flowering time or metal tolerance are allowed to change. What do you think would happen if flowering time was allowed to change, but metal tolerance was not? Briefly explain your prediction.
Question 8.2.5
What do you think would happen if metal tolerance was allowed to change, but flowering time was not? Briefly explain your prediction.
8.3. Looking at Flowering Time
|
Set the initial values to:
|
-
Press SETUP. and Press GO/STOP to run the model.
-
You can switch the VISUALIZE-TIME-STEPS to “years” or “days”. Years runs faster, but days lets you see the actual difference (if any) in flowering times between plants.
-
Run, the model for at least a hundred years.
-
Analyze the FLOWER-TIMES graph.
-
Record your data in your Observation section.
Question 8.3.1
Observations
Question 8.3.2
Were your predictions correct? Explain.
Question 8.3.3
What is the range of flower times?
Question 8.3.4
Let's look at why the flowering times show this pattern. Which type of individual would have the best chances of being pollinated by other flowers?
A plant with a flowering time much later than most of the population
A plant with a flowering time similar to most of the population
Question 8.3.5
How does the shape of the FLOWER-TIME graph from this model run support this claim: "Any individual that flowers earlier than the average flower time or later than the average flower time, will have a lower chance of having offspring?"
Question 8.3.6
Do you think flowering time is under stabilizing selection or directional selection? Explain your choice.
Question 8.3.7
All of the plants are flowering in a very narrow range of flowering times, opening during a similar time of year. Do you think all of the plants are still part of the same species? Why or why not?
8.4. Looking at Metal Tolerance
|
Set the initial values to:
|
-
Press SETUP. and Press GO/STOP to run the model.
-
You can switch the VISUALIZE-TIME-STEPS to “years” or “days”. Years runs faster, but days lets you see the actual difference (if any) in flowering times between plants.
-
Run, the model for at least a hundred years.
-
Analyze the FLOWER-TIMES graph.
-
Record your data in your Observation section.
Question 8.4.1
Observations
Question 8.4.2
Were your predictions correct? Explain.
Question 8.4.3
What is the range of metal tolerance values?
Question 8.4.4
Metal tolerance is inherited from both parent plants. Knowing that all of the plants have the same flowering time, why do you think the plants mostly have similar metal tolerances, even when they are in the blue region?
Question 8.4.5
The plants in the left region and the plants in the right region tend to have different metal tolerances. Do you think they are still part of the same species? Why?
8.5. Predict
Purpose
Where do new species come from?
Predict
In the next model run you will allow both flower time mutations and metal tolerance mutations to occur in the offspring.
Question 8.5.1
What do you predict the outcome will be for the metal tolerance of the plants?
Question 8.5.2
What do you predict the outcome will be for the flower time of the plants?
8.6. Test Your Predictions
|
Set the initial values to:
|
-
Press SETUP. and Press GO/STOP to run the model.Keep the model running until the SIMULTANEOUS FLOWERING graph shows that the number of simultaneously flowering plants in the contaminated soil and in the regular soil, is very close to zero.
-
Now make sure you have switched back VISUALIZE-TIME-STEPS to “days” mode and keep the model running.Below, record what you notice about when the flowers on the left side of the ecosystem are blooming versus the flowers on the right side of the ecosystem.
In the next set of questions you will try to explain why the flowers on the right have a different flower time than those on the left. To do this you may want to rerun this previous exploration in “days” mode, change labels, and study the model graphs. Feel free to conduct new experiments in this exploration to help you understand and explain why the initial plant population has “speciated”.
Question 8.6.1
Observations
Question 8.6.2
Why are the tolerance values in the two regions different, and why do you think there aren't there many plants in the population with a tolerance in between 10 and 90? Think back to the concepts of fitness, natural selection, and adaptation in response to different environments from the previous lessons.
Question 8.6.3
When the plants first reached the blue contaminated soil, the plants growing in that region increased their metal tolerance very quickly. Do you think this was a result of stabilizing selection or directional selection? Why?
Question 8.6.4
Now let's think about the differences in flowering times. If a plant with no metal tolerance, growing on the left side (clean soil) were to reproduce with a plant with metal tolerance growing on the right side (contaminated soil), their offspring would inherit genetic information from both parents. Why would this offspring plant be at a competitive disadvantage for survival compared to other plants growing either in the clean soil or in the contaminated soil?
Question 8.6.5
If a plant has to reproduce with another plant in order to have offspring, why would flowers in metal soil evolve a different average flower time than the flowers in the clean soil?
Question 8.6.6
| The type of selection acting on flowering times, where intermediate values are selected against and variation increases, is called disruptive selection. How is it similar to or different from stabilizing and directional selection? |  |
Question 8.6.7
How do the TOLERANCES and FLOWER TIMES graphs support the claim that: "The population of plants on the left side of the ecosystem no longer breed with the population of plants on the right side of the ecosystem"
Question 8.6.8
Do you think that the population of plants on the left side and the population of plants on the right side are still part of the same species? Why or why not?
8.7. Discoveries and Insights
Where do new species come from?
Question 8.7.1
What is the one big idea that you learned that helps you answer the question above?
Question 8.7.2
Do you think that the process you observed here will be similar for animal populations? Explain.
Question 8.7.3
How did this lesson inform or give new insights about what has happened in the Galapagos?









